In this post I’m going to look at completing the square, a fairly tricky topic in GCSE maths. Many students learn the procedure but don’t quite know why they are doing it.
Completing the square is useful because it enables us to solve quadratic equations that do not factorise easily and also helps to identify its maxima and minima without the need to draw a graph (not discussed here).
Take a look at this quadratic equation:

Attempting to solve this by factorisation (double brackets method) is difficult as you can only factorise easily if the discriminant is a perfect square.
As you can see below, the discriminant is not a square number:
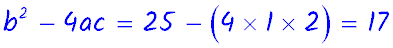
So, there are two other methods we can use instead. The first is to use the quadratic formula:
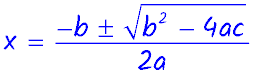
The other method, is to use the method of completing the square:
Step 1
Using the same quadratic equation,

take out an x, and add it to half of the coefficient of the second term:

Step 2
Square the result of step 1.
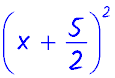
At this stage, it is useful to know what exactly we have done. If you expand the above, you get:

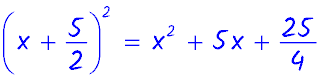
Compare this to the original quadratic equation:
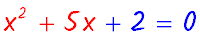
The bit I have highlighted in red is the same, but with the fraction removed:
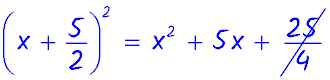
Step 3
Subtract the square of the second term in the bracket:
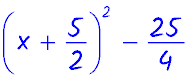
Step 4
All we have to do now is not forget to add the 2. We will then have converted the original quadratic expression into an alternative form which is easier to solve.
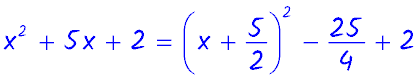
Therefore, we have:
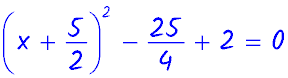

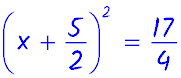
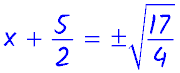
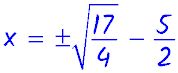

So, that is how you can solve a quadratic equation using the completing the square method. Please see my YouTube video for more on completing the square: